Modified block compressed sparse row (MBCSR) format.
An 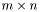 matrix
matrix 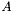 stored in
stored in 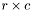 MBCSR format is logically partitioned row-wise into 3 submatrices,
MBCSR format is logically partitioned row-wise into 3 submatrices,  , where
, where 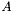 is stored in
is stored in 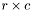 MBCSR format. This partitioning is a `canonical' format in which
MBCSR format. This partitioning is a `canonical' format in which 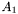 ,
, 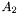 , and
, and 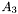 have the following properties:
have the following properties:
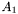 consists of all block rows and diagonal blocks of size
consists of all block rows and diagonal blocks of size 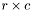 and
and 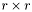 , respectively.
, respectively.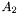 contains the degenerate diagonal block of size
contains the degenerate diagonal block of size 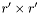 where
where 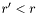 , and the corresponding block row of size
, and the corresponding block row of size 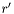 that contains it.
that contains it.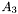 contains no diagonal blocks (i.e., is simply stored in BCSR format).
contains no diagonal blocks (i.e., is simply stored in BCSR format).
The purpose of this format is to enable fast implementations of the triangular solve and 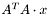 kernels in which the `special case' code to handle degenerate diagonal block, if any, is stored separately in
kernels in which the `special case' code to handle degenerate diagonal block, if any, is stored separately in 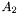 , and multiplication by
, and multiplication by 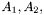 and
and 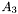 can execute at `full' speed. The canonical form provides a uniform way to treat both square and rectangular matrices stored in MBCSR format.
can execute at `full' speed. The canonical form provides a uniform way to treat both square and rectangular matrices stored in MBCSR format.
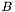 stored in
stored in 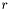 divides
divides 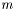
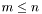
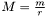 be the number of block rows. Then
be the number of block rows. Then 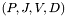 , as follows:
, as follows: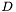 is an array of length
is an array of length 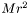 containing all the elements of
containing all the elements of 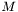 diagonal blocks beginning at element
diagonal blocks beginning at element 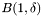 where each block is a square
where each block is a square 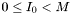 ,
, 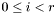 , and
, and 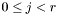 , the array element
, the array element ![$D[I_0\cdot r^2 + i\cdot r + j]$](form_157.png) stores the matrix element
stores the matrix element 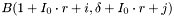 .
.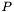 is an integer array of block-row pointers, of length at least
is an integer array of block-row pointers, of length at least 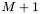 .
.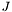 is an integer array of block column indices, of length at least
is an integer array of block column indices, of length at least ![$P[M]$](form_27.png) .
.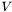 is an array of non-zero block values, of length at least
is an array of non-zero block values, of length at least ![$P[M]\cdot r\cdot c$](form_29.png) .
.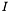 , where
, where 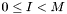 , as rows
, as rows 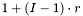 through
through 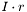 of
of 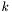 such that
such that ![$P[I-1] \leq k \leq P[I]$](form_35.png) ,
, ![$j_0=J[k]+1$](form_36.png) is the column index of the
is the column index of the 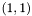 entry of an explicitly stored non-zero block whose values are laid out in row-major order in the subarray
entry of an explicitly stored non-zero block whose values are laid out in row-major order in the subarray ![$V[krc : (k+1)rc-1]$](form_38.png) .
.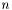 of
of 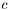 does not divide
does not divide 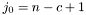 . If such a block overlaps with another block starting at column index
. If such a block overlaps with another block starting at column index 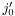 , then the initial columns of the block at
, then the initial columns of the block at 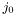 are set to zero.
are set to zero.  1.4.6
1.4.6